PIMS - UCalgary Geometric Analysis Seminar Series: Diego Maldonado
Topic
Speakers
Details
Certain convex functions on $R^n$ produce quasi-distances and Borel measures on $R^n$. Furthermore, their Hessians can shape degenerate/singular elliptic operators. We will describe geometric and measure-theoretic conditions on convex functions that allow to prove a Harnack inequality for nonnegative solutions to their associated elliptic operators.
Speaker Biography: Diego Maldonado is a Professor in the analysis group in the Department of Mathematics at Kansas State University. He researches singular elliptic PDEs, with a focus on regularity estimates for the Monge-Ampère equation and singular Calderón-Zygmond integrals.
Additional Information
This seminar is available via Zoom. Registration is required.
Time: 2–3pm PST (3–4pm MDT)
-------
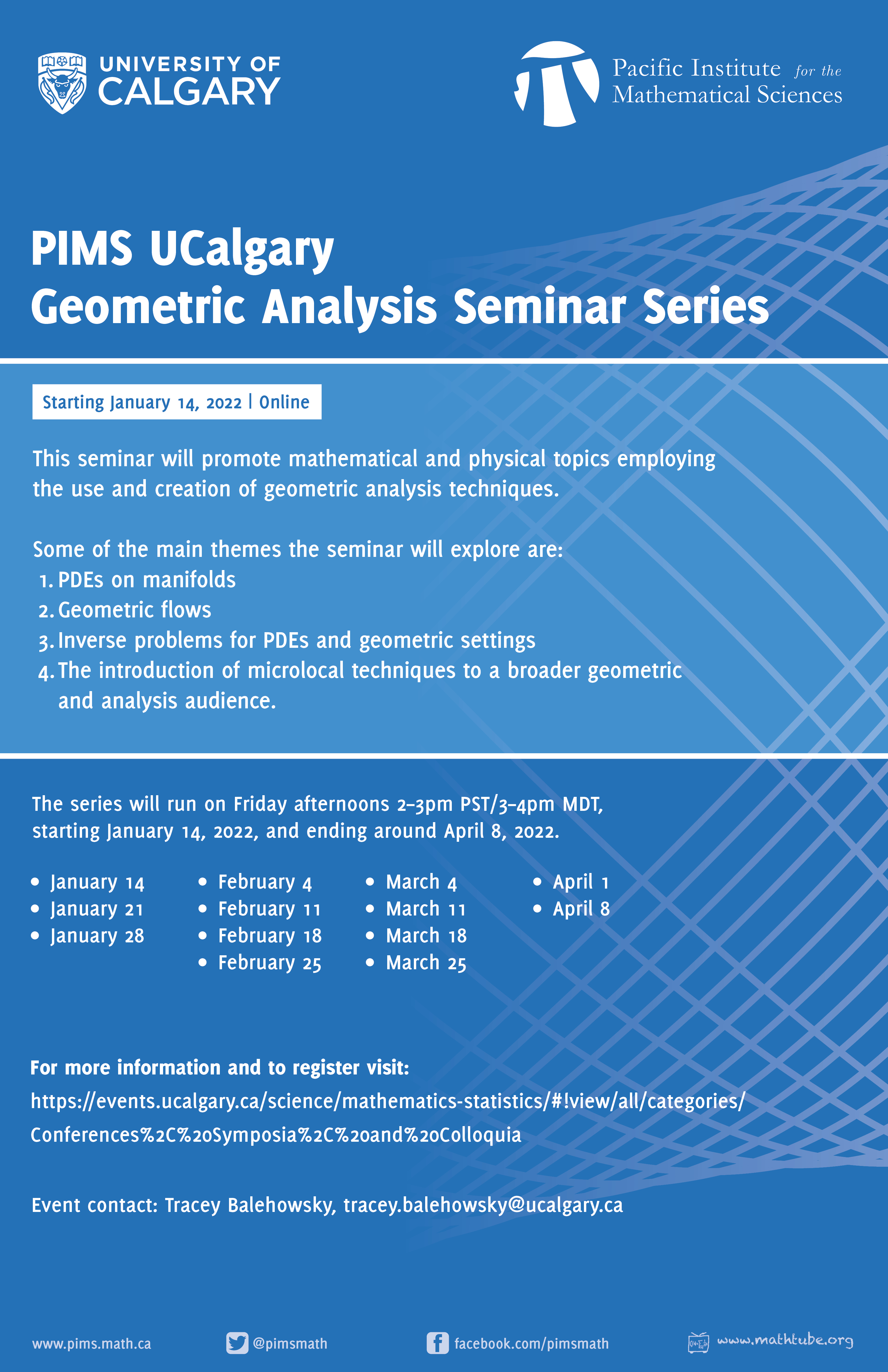
This seminar series will promote mathematical and physical topics employing the use and creation of geometric analysis techniques. Some of the main themes the seminar will explore are 1) PDEs on manifolds, 2) geometric flows, 3) inverse problems for PDEs and geometric settings, and 4) the introduction of microlocal techniques to a broader geometric and analysis audience.
The series will run on Friday afternoons 2–3pm PST (3–4pm MDT), starting January 14, 2022 and ending around April 8, 2022. See more information and other dates in the series or contact Tracey Balehowsky, tracey.balehowsky@ucalgary.ca
Diego Maldonado, Kansas State University